The brief itself was to utilise numbers to be the overall subject-matter and driver of an experimental website. Free-reign was granted in the interpretation of what that entails. The website had to contain five unique pages, each tackling a different subject or approach to interpretation, an about page detailing what the site is about.
Web Design, Motion Graphics, Editorial Design, Graphic Design, HTML, CSS, Js, After Effects, Trapcode, 3D Modelling
Mathematics is more often than not the bane of students and young adults everywhere, creating dread rather than intrigue at the tools that it gives us and the tremendous capabilities it possesses.
Numbers is a website geared towards illustrating some of the most complicated mathematical concepts, theorems, and formulas in beautiful, abstract, and artistic ways by turning them into the driving force for motion graphics. Each section takes on a different concept and is consequently visualised in accordance with what unique aspect of the concept makes it so powerful, versatile, or interesting. To provide some context to each section, a breakdown in the theoretics is provided alongside it.
The home page is kept simple and clean, just a logo, an "about" button, all the section links, and a describer of the brief as a whole.

The "about" section essentially gives the reason why the brief was approached in such an abstract way, but also gives viewers a hint of what's to come.

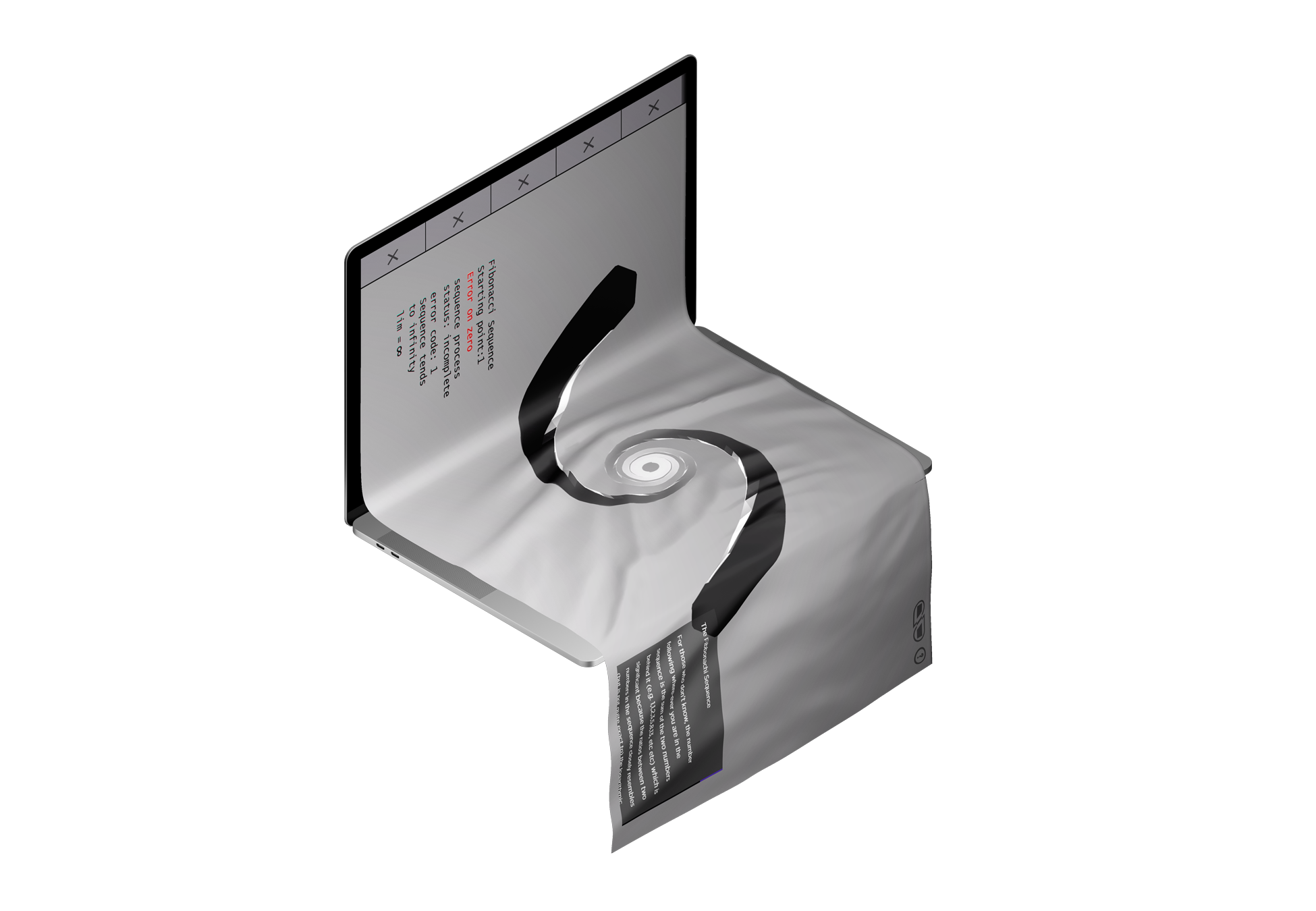
Section 1 is all about the Fibonnacci sequence and its potential for interesting pattern generation. The Fibonnacci sequence is a sequence of numbers that in any part of the sequence, the combination of two numbers results in, without fail, the next number in the sequence ad infinitum. It often is seen in the natural world in the form of perfect spirals, hence the motion motion-graphic following that form

Section 2 is all about pi, a ratio that has incredible significance to geometry, astronomy and architecture. The ratio is always a fixed, irrational number regardless the size of the circle and as such, is considered one of the most significant mathematical constant expressions in the world, of which, few actually exist.

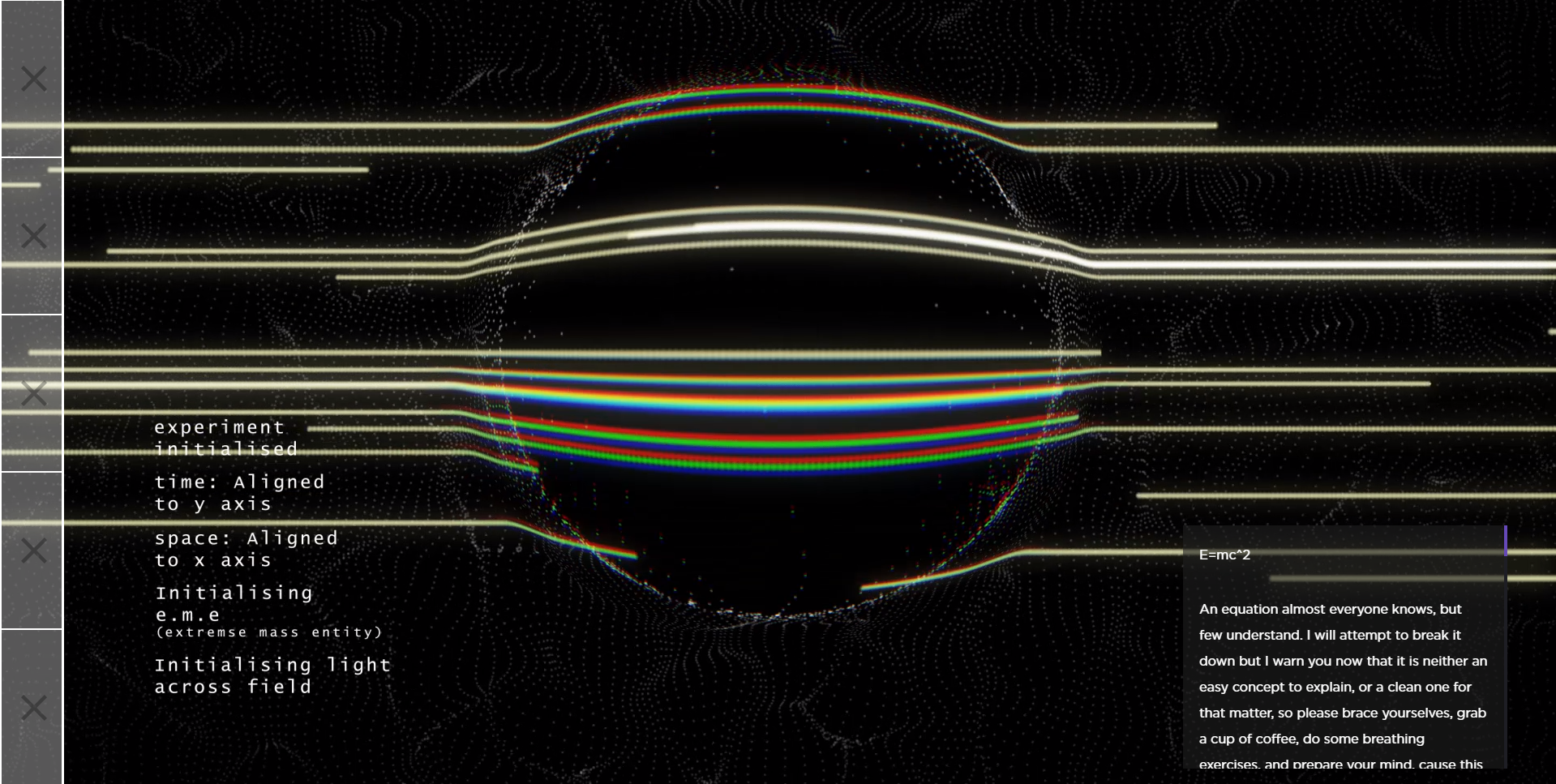
Section 3 is about a theoretical framework that many of us know but few understand. The General Theory of Relativity posits that gravity is not a force in the same sense as Newton first suggested, but rather space-time bends in response to it. We all know that light bends in response to the gravitational pull of black holes, which shouldn't be possible, because light has no mass. The only explaination is that gravity bends the space that light is travelling through, thus proving Einsteins theory correct. The motion-graphic associated with this section is just to illustrate the point .
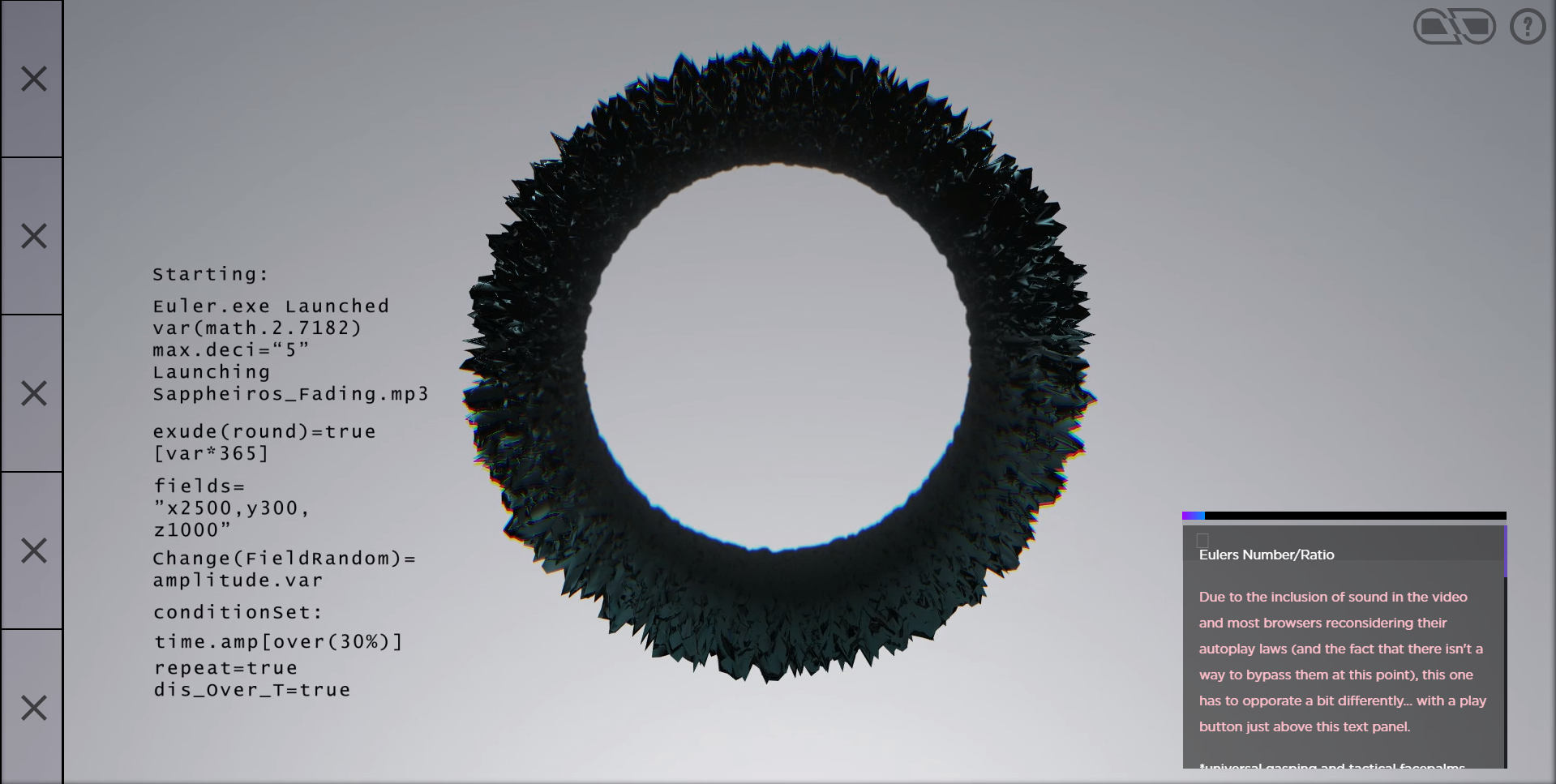
Section 4 is about Euler's Number, which is considered the ideal ratio in financial maths. It’s importance is most of associated with the point before which the increase in number of times
interest is compounded while the interest is divided into half its original value, reaches a point before any further divisions will result in diminishing returns.
But this doesn’t only occur in financial maths;
it turns out that by chance, many things hold Euler’s Number as the median guiding point before diminishing returns can occur. It is thus considered the ultimate median point. The way we here music is dependant
on frequencies resonating the air at specific intervals, perfect notes are as such, also dependant on perfect medians
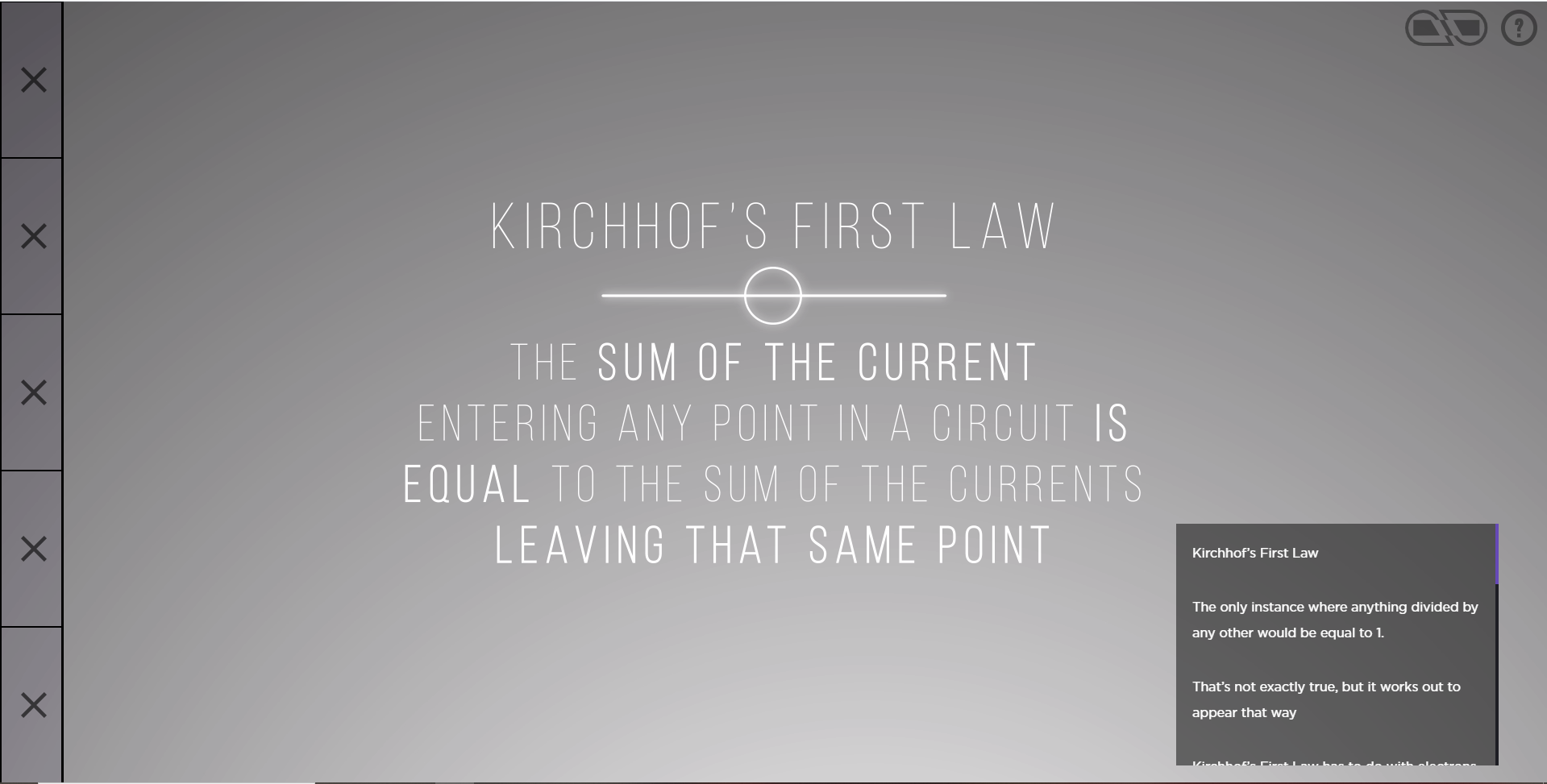
Section 5 is about Kirchhof’s First Law, which has to do with electrons and the conservation of current in a closed system, provided that there is no change in charge. The sum of current entering any point in the circuit should remain the same as the sum of current exiting the circuit. It is one of the only physical instances of anything divided by any other would always be equal to 1. This logical divergence between maths and science is integral to the opperation of all electronics in the world, including the device you're reading this on.